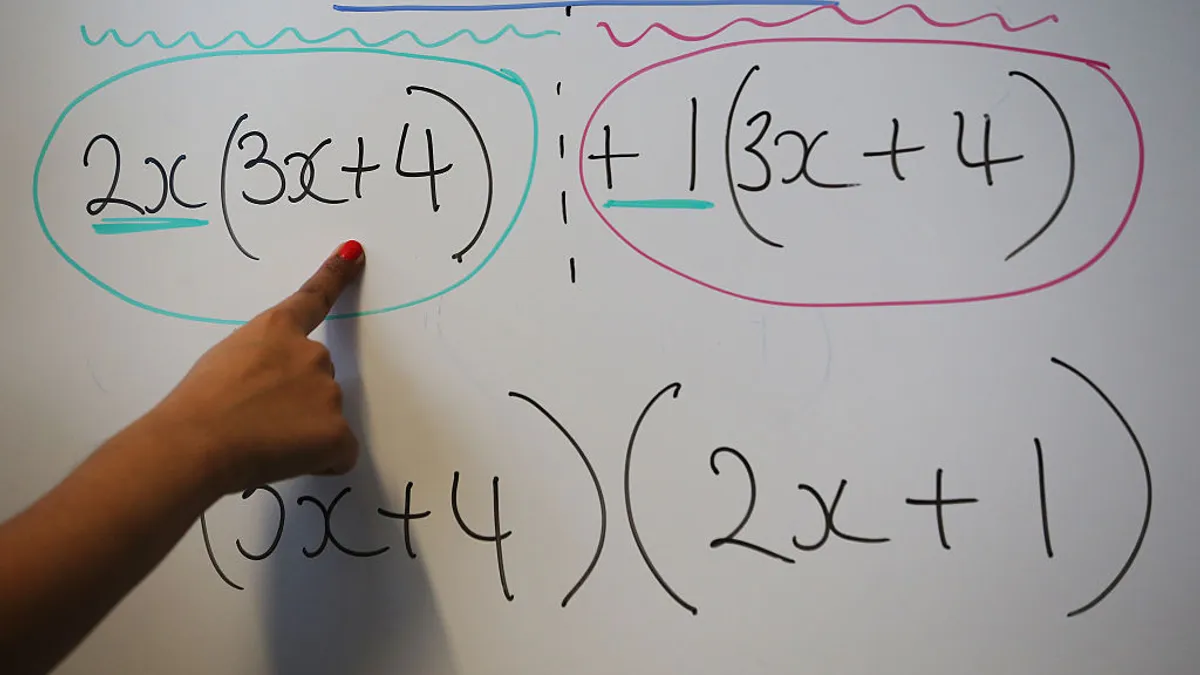Dive Brief:
-
As the “science of reading” gains more traction from states, there is interest in revamping the way mathematics is taught to students as part of a movement to adopt a “science of math” approach.
-
But this method, while couched as a novel model, is not new but is rooted in tools used for decades and often with little success, said Kevin Dykema, president of the National Council of Teachers of Mathematics.
-
“There isn’t a focus on understanding the math, but a focus on the correct answer,” Dykema said. “That has not worked in the past.”
Dive Insight:
To Dykema, the primary focus for the “science of math” approach is on procedural fluency, or the ability to apply the processes of how to solve problems and build to the next level. But NCTM’s president said procedural fluency is just one of five strands needed to help students master and grow proficient in math.
At the top of those strands is conceptual understanding, when students begin to answer the age-old question, “Where will I ever use this?” While procedural fluency is important, the remaining three strands — adaptive reasoning, strategic competence and productive disposition — are also critical in helping to anchor mathematic reasoning and skill-building, Dykema said.
“We need to focus on all five strands,” Dykema said. “We don’t want to just focus on procedural fluency. We want to consider how [students] feel as mathematicians. Do they feel capable of learning math? Or, to them, is this a worthless subject?”
Dykema said a teacher-driven model, where educators model how to solve a problem and then have students answer math sets, can lead to declines in math test scores. This style of math education where students perform computations without knowing the purpose behind them is in the majority of classrooms today, he said.
“It’s giving a new name to something that's been done for many decades,” Dykema said.
Instead, as technology — especially artificial intelligence — advances, Dykema suggests that knowing how to perform specific tasks such as calculations is less critical than being able to apply mathematical understanding.
“We want them to understand the math facts but also how they are getting to them," he said.